Simplification of Numerical Expressions
This topic would discuss on simplification of numerical expression using BODMAS rule. The word BODMAS stands for:
B stands for Brackets
O stands for Of
D stands for Division
M stands for Multiplication
A stands for Addition
S stands for Subtraction
Now in brackets there are three sub categories:
( ) ……. First bracket
{ }……..Second bracket
[ ] ……...Third Bracket
In this bracket classification there is certain rule. The rule is that the operation in first bracket has to be performed first then the operation in second bracket and finally the operation in third bracket.
Here are few examples on Simplification of numerical expressions:
1. 12 + [2 { 13 ÷ (50 + 15)}]
Solution:
12 + [2 {13 ÷ (50 + 15)}]
= 12 + [2 {13 ÷ 65}] The first bracket is solved first
= 12 + [2 × 0.2] The operation of the second bracket is done
= 12 + 0.4
= 12.4
Explanation:
We can see that under first bracket 50 and 15 is first added to get 65. Hence the operation of the first bracket is over. Then 13 is divided by 65 to get 0.2 and hence we can say that the operation of the second bracket is done. Then 2 is multiplied with 0.2 to get 0.4 which involves completion of the operation of third bracket. Now finally 12 is added to 0.4 to get 12.4 which is the final answer.
2. 56 + 4 × 2 – ( 30 ÷ {18 – 3})
Solution:
56 + 4 × 2 – { 30 ÷ (18 – 3)}
= 56 + 4 × 2 – {30 ÷ 15} Operation of first bracket performed
= 56 + 4 × 2 – 2 Operation of second bracket performed
= 56 + 8 – 2
= 64 – 2
= 62
Explanation:
We can see in this problem that there are only two brackets first bracket and second bracket. Therefore, we have to perform the operation of the first bracket and then second bracket. Under the first bracket we have to first perform subtraction that is 3 subtracted from 18 we get 15. Then under second bracket 30 is divided by 15 to get 2. Then we have three more operations to perform that is addition, multiplication and subtraction. So according to BODMAS rule we have performed first multiplication then addition and lastly subtraction to get the final result as 62.
3. 15 + 5 × (115 × 0) + 20 – (15 ÷ 3)
Solution:
15 + 5 × (115 × 0) + 20 – (15 ÷ 3)
= 15 + 5 × 0 + 20 – 5 Operation of first bracket performed
= 15 + 20 – 5
= 35 – 5
= 30
Explanation:
Here in this sum we only have first bracket but there are two first brackets. These two first brackets have to be solved in same step only. After solving the operation in two first brackets we have to carry out the other operations like addition, multiplication, and subtraction. First multiplication is done then addition and lastly subtraction according to BODMAS rule.
From Simplification of Numerical Expressions to HOME PAGE
Recent Articles
-
Explain about Growth in Plants |Definition of Growth & Differentiation
Feb 27, 25 02:07 PM
Growth is a permanent increase in length or volume of an organism that brought upon by an increase in its dimensions due to synthesis of new protoplasmic material. -
Definition of Respiratory Quotient | calculation | Application | Plant
Dec 02, 24 12:09 AM
Definition of respiration quotient- the ratio of the carbon-dioxide evolved to that of the oxygen consumed by a cell, tissue, plants or animals in a given time is called respiratory quotient. It is us… -
Amphibolic Pathway | Definition | Examples | Pentose Phosphate Pathway
Jun 06, 24 10:40 AM
Definition of amphibolic pathway- Amphibolic pathway is a biochemical pathway where anabolism and catabolism are both combined together. Examples of amphibolic pathway- there are different biochemical… -
Respiratory Balance Sheet | TCA Cycle | ATP Consumption Process
Feb 18, 24 01:56 PM
The major component that produced during the photosynthesis is Glucose which is further metabolised by the different metabolic pathways like glycolysis, Krebs cycle, TCA cycle and produces energy whic… -
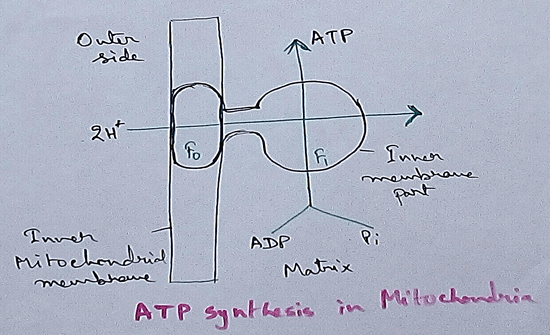
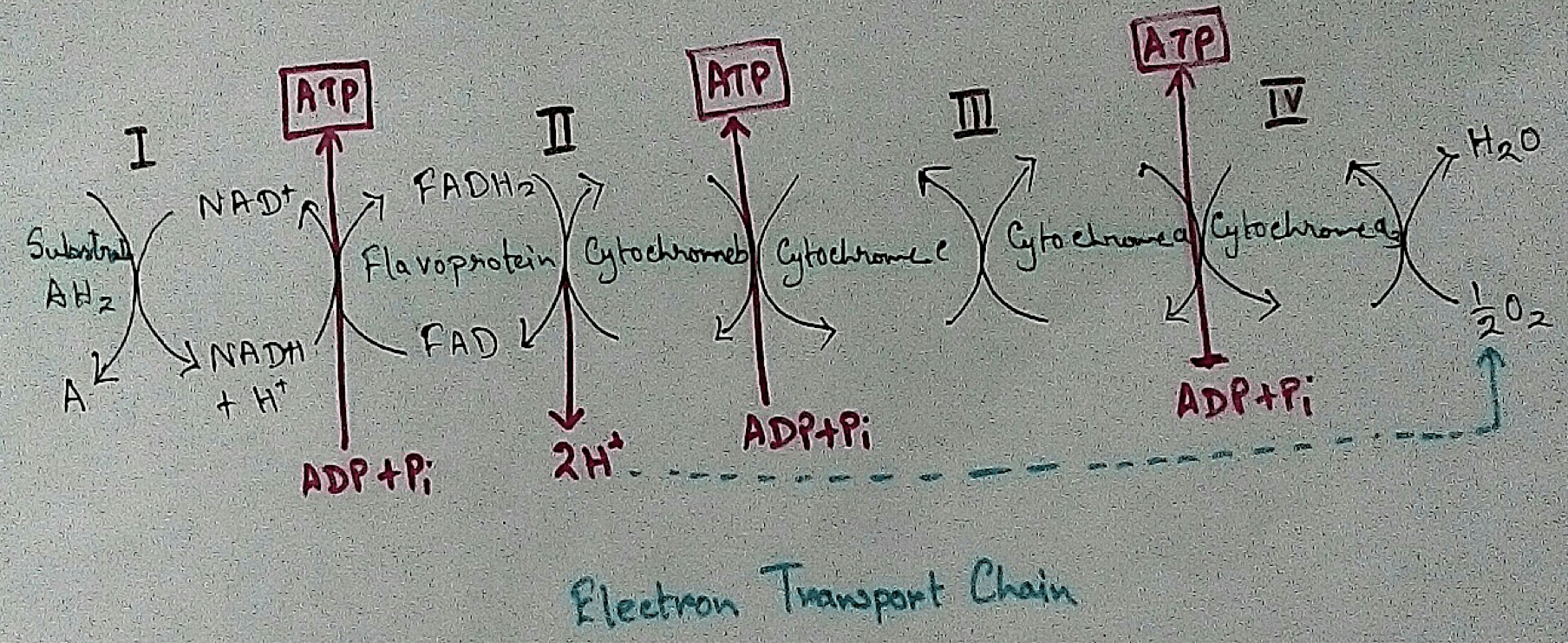
Electron Transport System and Oxidative Phosphorylation | ETC |Diagram
Feb 04, 24 01:57 PM
It is also called ETC. Electron transfer means the process where one electron relocates from one atom to the other atom. Definition of electron transport chain - The biological process where a chains…



New! Comments
Have your say about what you just read! Leave me a comment in the box below.