Relationship between H.C.F. and L.C.M.
This topic discusses about the relationship between H.C.F and L.C.M in case of two numbers. The relationship between H.C.F and L.C. M of two number states that:
H.C.F × L.C.M = First Number × Second Number
Hence from this relationship we can find the following:
\[\textrm{H.C.F} = \frac{\textrm{First Number} \times \textrm{Second Number}}{\textrm{L.C.M.}}\]
\[\textrm{L.C.M.} = \frac{\textrm{First Number} \times \textrm{Second Number}}{\textrm{H.C.F.}}\]
\[\textrm{First Number} = \frac{\textrm{H.C.F.} \times \textrm{L.C.M.}}{\textrm{Second Number}}\]
\[\textrm{Second Number} = \frac{\textrm{H.C.F.} \times \textrm{L.C.M.}}{\textrm{First Number}}\]
To Justify this Relation here is an Example:
1. Suppose the two given numbers are 24 and 16. Justify the relationship between H.C.F and L.C.M
Solution:
The H. C.F of 24 and 18 by finding factors is:
The factors of 24 = 2 × 2 × 2 × 3 = 2^3×3
The factors of 18 = 2 × 3 × 3 = 3^2×2
The common prime factors of 24 and 18 = 2 and 3
The lowest power of 2 is 2
The lowest power of 3 is 3
Therefore, the Highest common factor (H.C.F) = 2 × 3 = 6
The L.C. M of 24 and 18 by prime factorization method:
The factors of 24 = 2 × 2 × 2 × 3 = 23 × 3
The factors of 18 = 2 × 3 × 3 = 32 × 2
The highest power of 2 = 23
The highest power of 3 = 32
Therefore, the lowest common multiple of 18 and 24 is 23 × 32 = 72
Now the product of H.C.F and L.C.M is 6 × 72 = 432
The product of 18 and 24 is 432
Hence, H.C.F × L.C.M = Product of two numbers
432 = 432 (verified)
Here are few more examples to illustrate relationship between H.C.F and L.C.M
2. The H.C.F of two numbers is 18 and their product is 94734. Find their L.C.M
Solution:
Given,
The highest common factor (H.C.F) of two numbers = 18
The product of two numbers = 94734
According to the relationship, we know,
H.C.F × L.C.M = First number × Second number
Or, H.C.F × L.C.M = product of the numbers
⟹ 18 × L.C.M = 94734
⟹ L.C.M = \(\frac{94734}{18}\)
⟹ L.C.M = 5263
Therefore, the lowest common multiple (L.C.M) of the two numbers = 5263
Explanation:
The H.C.F of two numbers is given and their product is given. By applying the relation we need to find out the L.C.M
3. The L.C.M and H.C.F of two numbers are 144 and 36 respectively. If one number is 54, then find the other number.
Solution:
Given,
The highest common factor (H.C.F) of two numbers = 36
The lowest common multiple (L.C.M) of two numbers = 144
One of the two numbers is 54
According to the relationship, we know,
H.C.F × L.C.M = First number × Second number
⟹ 36 × 144 = 54 × Second number
⟹ 5184 = 54 × Second number
⟹ Second Number = \(\frac{5184}{54}\)
⟹ Second Number = 96
Therefore, the other number is 96
Explanation:
The H.C.F and L.C.M of two numbers is given and one of the numbers is given. By applying the relationship we can find out the other/ second number.
From Relationship between H.C.F. and L.C.M. to HOME PAGE
Recent Articles
-
Eleventh Grade | Eleventh Grade Science | Eleventh Grade Math
Jun 27, 25 12:26 AM
Eleventh grade biology has been designed in accordance with the recommended topics. We will cover all the topics in biology very exciting and interesting way. -
Explain Digestion of Food | Salivary Glands | Oesophagus | Stomach
Jun 27, 25 12:20 AM
Before the digestion is start by the different enzymes secreted from the different digestive glands food must be turned and chut or mixed with saliva inside the mouth. -
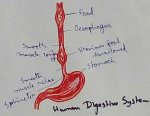
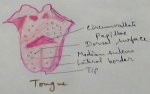
Explain Human Digestive System | Mouth | Tongue | Pharynx | Teeth
Jun 21, 25 01:15 PM
Digestive system is a system of alimentary canal and digestive glands. Alimentary canal- alimentary canal is a tube of variable diameter having muscular wall and glandular epithelial tissues which sta… -
Vernalisation in Plants | Definition | Mechanism | Devernalization |
Jun 18, 25 01:34 PM
Definition of vernalisation- The change of flowering habit due to the low temperature treatment is known as vernalisation. This is a physiological process which was denoted by Clipart in 1857 invite b… -
The Food We Eat | Food we Get from Plants and Animals | Carbohydrates
Jun 15, 25 03:20 PM
What are the food that we should eat? Find out the names of ten food items in the word maze. Write the names in the correct column of the table given below. Food we get from plants Food we get from an…



New! Comments
Have your say about what you just read! Leave me a comment in the box below.