Comparison of Decimal Fractions
This topic would deal with comparing decimal fractions. For comparing decimal fractions we first compare the whole number part then compare the fractional part. For comparing the fractional part we first compare the tenths place, then hundredths place, then thousandths place and so on.
For example:
Compare 0.55 and 0.58
We cannot compare the whole number part as both are same
We are comparing the fractional decimal part
0.55 = 5 tenths + 5 hundredths
0.58 = 5 tenths + 8 hundredths
Now 5 tenths = 5 tenths
We will now compare next place
5 hundredths < 8 hundredths
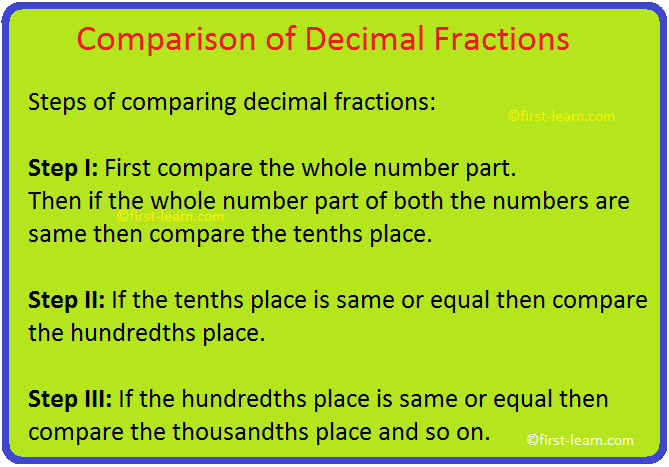
Steps of comparing decimal fractions:
Step I: First compare the whole number part.
Then if the whole number part of both the numbers are same then compare the tenths place.
Step II: If the tenths place is same or equal then compare the hundredths place.
Step III: If the hundredths place is same or equal then compare the thousandths place and so on.
Here are few examples of comparing decimal fractions:
1. Compare 45.63 and 45. 976
45.63 = 4 tens + 5 ones + 6 tenths + 3 hundredths
45.976 = 4 tens + 5 ones + 9 tenths + 7 hundredths + 6 thousandths
4 tens = 4 tens
5 ones = 5 ones
6 tenths < 9 tenths
Therefore, 45.63 < 45.976
2. Which is greater 90.678 or 65.321?
90.678 = 9 tens + 0 ones + 6 tenths + 7 hundredths + 8 thousandths
65.321 = 6 tens + 5 ones + 3 tenths + 2 hundredths + 1 thousandths
Now we see,
9 tens > 6 tens
Hence 90.678 >65.321
3. Compare 45.213 and 785. 563
45.213 = 4 tens + 5 ones + 2 tenths + 1 hundredths + 3 thousandths
785.563 = 7 hundreds + 8 tens + 5 ones + 5 tenths + 6 hundredths + 3 thousandths
Now we will first compare the integral part
4 tens < 7 hundreds
Therefore, 45.213 < 785.563
4. Which is greater 32.124 or 32.128
32.124 = 3 tens + 2 ones + 1 tenths + 2 hundredths + 4 thousandths
32.128 = 3 tens + 2 ones + 1 tenths + 2 hundredths + 8 thousandths
Now we will first compare the integral part
3 tens = 3 tens
2 ones = 2 ones
Now we will compare the decimal fraction part
1 tenths = 1 tenths
2 hundredths = 2 hundredths
4 thousandths < 8 thousandths
Therefore, 32.124 < 32.128
You might like these
Worksheet on Divisibility Rules | Questions on Test of Divisibility
This is a worksheet which will provide few problems on the divisibility rule of 2, 3, 4, 5, 6, 7, 8, 9, and 10. 1. Check whether the following numbers are divisible by 2 or 3 or both? (i) 2562 (ii) 5693 (iii) 2201 (iv) 7480 (v) 5296 (vi) 4062 (vii) 4568 (viii) 1425 (ix) 1110
Problems on Divisibility Rules | Rules to Test of Divisibility | Test
Here are few problems on the divisibility rules of 2, 3, 4, 5, 6, 7, 8, 9, and 10 which will help the learners in revising their concepts on the divisibility rules. 1. Check whether 3456 is divisible by 2? Solution: The last digit is an even number (i.e. 6) hence 3456 is
Divisible by 10|Test of Divisibility by 10|Rules of Divisibility by 10
The divisibility rule of 10 states that If the last digit of a number is 0 then the given number is divisible by 10. For eg: Check whether 5400 is divisible by 10 or not? Solution: As the last digit of the number is 0 hence, 5400 is divisible by 10
Divisible by 9 | Test of Divisibility by 9 |Rules of Divisibility by 9
This rule states that a number is divisible by 9 if the sum of its digits of the number is divisible by 9 For eg: Check whether 729 is divisible by 9 or not? Sum of the digits = 7 + 2 + 9 = 18 Now 18 is divisible by 9 hence, 729 is also divisible by 9 Here are few examples
Divisible by 8 | Test of Divisibility by 8 |Rules of Divisibility by 8
The most common method to check whether a number is divisible by 8 is to divide and see if the quotient is a whole number or not? If the quotient is a whole number then the given number is divisible by 8. But there is an easier way to check by using the divisibility rule.
Divisible by 7 | Test of Divisibility by 7 |Rules of Divisibility by 7
This rule states that if the difference between twice the digit at units place and the number formed from the remaining digits of the given number is divisible by 7 then the whole number is divisible by 7.
Divisible by 6 | Test of divisibility by 6 |Rules of Divisibility by 6
This topic on divisible by 6 will discuss about the divisibility rule of 6 and few example on the same. Divisibility rule of 6: A number is divisible by 6 if the prime factor of that is 2 & 3 is divisible by 6. For Example: 216 216 is divisible by 2 as the last digit 6 is
Divisible by 5 | Test of divisibility by 5 |Rules of Divisibility by 5
This topic on divisible by 5 will discuss on the divisibility rule of 5 and illustrate few example on the same. Divisibility Rule of 5: If a number ends with 0 or 5 then the number is divisible by 5. For Example: 550 Since the number ends with 0 hence the number is divisible
Divisible by 4 | Rules of Divisibility by 4|Divisibility Rules & Tests
This topic i on divisibility rule of 4 and will provide examples on the same. Divisibility Rule of 4: If the last two digits of the number is divisible by 4 then the whole number is divisible by 4 For eg: 524 The last two digit of the number is 24 which is divisible by 4.
From Comparison of Decimal Fractions to HOME PAGE
Recent Articles
-
Mechanism of Breathing | Definition of Inspiration and Expiration
Aug 17, 25 11:41 PM
Breathing is the process which is accomplished in three states that is inspiration expiration and pause . Definition of inspiration - Entry of air into the lungs from outside during breathing is calle… -
Human Respiratory System | External Nares | Nasal Cavity | Pharynx
Aug 04, 25 03:14 PM
Definition of respiration - This is the process of making energy available to organisms and their living cells through enzyme controlled catabolic breakdown of organic molecules. The organic materials… -
Disorders of Digestive System | Symptoms of Jaundice | Vomiting |
Jul 16, 25 12:18 PM
Jaundice- It is a disease that occurs due to Umesh discoloration of the skin due to deposition of bilirubin and biliverdin pigment. Jaundice can be offered according to the different position like pre… -
Absorption of Digested Products | Absorption of Water | Nephrons
Jul 09, 25 02:24 PM
Food and water is observed in different parts of the body and is distributed in different cells and tissues. Absorption of food is observed to be observed in the small intestine in the specific type o… -
Eleventh Grade | Eleventh Grade Science | Eleventh Grade Biology
Jun 27, 25 12:26 AM
Eleventh grade biology has been designed in accordance with the recommended topics. We will cover all the topics in biology very exciting and interesting way.
New! Comments
Have your say about what you just read! Leave me a comment in the box below.