Integers

Integers are similar to whole numbers but they include negative numbers and just like whole numbers do not include any fractional part in them. Integers are represented with capital letter (Z) it is denotes Zahlen German word. Integers include positive numbers (without fractional part), negative numbers (without fractional part) and zero.

Integers can be represented as = {…....... -8, -7, -6, -5, -4, -3, -2, -1, 0, 1, 2, 3, 4, 5, 6, 7, 8 ……..}

Therefore -8, -7, -6, -5, -4, -3, -2, -1, are negative integers and positive integers include 1, 2, 3, 4, 5, 6, 7, 8. 0 is a neutral integer that is neither positive nor negative.

Whereas, other numbers like \(\frac{3}{7}\), √5, (\frac{17}{9}\) are not integers.

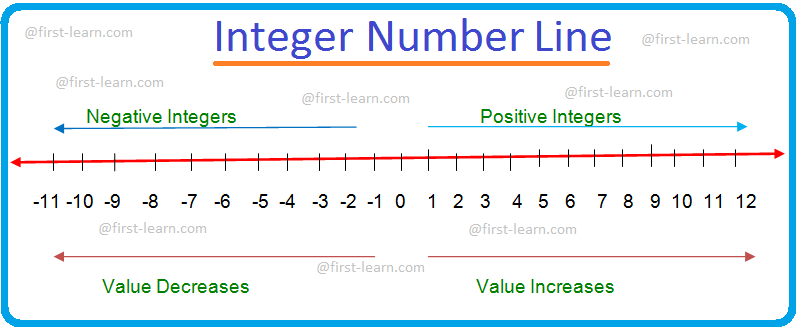
Integers can be represented with the help of a number line:

Properties of Integers:

Note: p, q and r are integers and q is a non-zero integer

Commutative property of integers

If we change the order of integer in addition the result remains unchanged

                             p + q = q + p

 

If we change the order of integer in subtraction the result changes

                             p – q ≠ q – p

If we change the order of integers in multiplication the result remains unchanged

                             p × q = q × p

 

If we change the order of integers in division the result changes

                             p ÷ q ≠ q ÷ p

 

Closure Property of integers

If we add two integers the result is an integer

                             p + q is an integer

 

If we subtract two integers the result is an integer

                             p – q is an integer

 

If we multiply two integer the result is an integer

                             p × q is an integer

 

If we divide two integer the result is necessarily not an integer

                             p ÷ q is necessarily not an integer.

 

Associative Property of Integers

If we change the grouping of integers in addition the result remains unchanged

                             (p +q) + r = p + (q +r)

 

If we change the grouping of integers in subtraction the result changes

                             (p –q) –r ≠ p – (q – r)

 

If we change the grouping of integers in multiplication the result remains unchanged

                             (p × q) × r = p × (q × r)

 

If we change the grouping of integers in division the result changes

                             (p ÷ q) ÷ r ≠ p ÷ (q ÷ r)

 

Distributive Property of Integers over Addition

In this property the integers are first added and then multiplied or first multiplied then added

                             p × (q + r) = p × q + p × r

Distributive Property of Integers over Subtraction

In this property the integers are first subtracted and then multiplied or first multiplied then subtracted.

                             p × (q - r) = p × q - p × r

 

Identity Property of Integers

This property states that if zero is added to any integer the result is the integer itself.

Additive identity = 0

p + 0 = p

This property also states that if zero is multiplied to any integer the result is zero and if 1 is multiplied with any integer the result is the integer itself. Moreover, if an integer is multiplied with -1 the result will be an integer itself but with an opposite sign.

Multiplicative identity = 1

p × 1 =p

p × 0 = p

From Integers to HOME PAGE